Issues in implementation

Student not progressing in Rocket Math Online Tutor? How to diagnose the problem. Part One

Posted on by Dr. Don

Progress in Rocket Math Online Tutor means passing levels in a Learning Track and then completing a Learning Track.  Students who are not progressing in Rocket Math will get discouraged.  The point of this educational app is for students to learn math facts by memory, by instant recall, instead of having to figure them out.  If students are not progressing, something is wrong.  Here’s the things to look for in order of how likely they are to be the problem.

Problem 1: Not completing sessions

Students can start a session and work for only a couple of seconds and then quit.  If students are not completing sessions then they are not doing enough practice.  They will not make progress unless they complete sessions regularly.

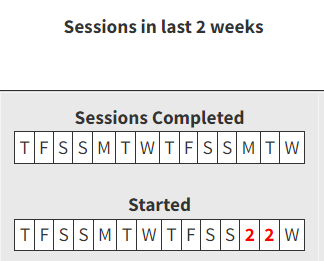
If you open the Review Progress tab and scroll down to the listing of your students you will see a column titled Sessions in last 2 weeks.  For each student, for the last two weeks, you can see how many sessions they completed each day as well as how many they started.  The data showing here tells you the student is starting sessions, but not completing them.  They are only ten minutes long, so they can do it if you tell them they should.

Solution 1: Require students to show Session Completed screen.

Rocket Math has a screen that proves students have completed their session each time they do Rocket Math. It comes up at the end of  the ten minute session.  This screen will stay up if they do not log out.  This is designed so you can require your students to show you they completed their session.  Because is will stay there, you can check them out (maybe use a clipboard?) and know they have completed their session.  Completing sessions is the first step in learning math facts and making progress.

Problem 2: Not working during sessions

Most students seem to understand that going as fast as they can with Rocket Math is the road to learning their math facts.  Most students like going fast and when Rocket Math helps them learn these facts they are motivated to develop mastery.  They realize it will make their lives easier when they have to do any arithmetic for any reason.

However, some students focus their efforts on trying to avoid learning and working. These student may log into Rocket Math but then go on to do something other than work on learning their facts.  These students will not spend much time practicing even when they are logged in.  They won’t of course, make much progress and that will be discouraging for both them and the teacher.

On the Review Progress screen, you can see each day how many problems students have answered in their ten-minute session. Student should be able to easily complete over 100 problems in a full ten-minute session, if they are working steadily.  Students who complete less than half that many problems are spending a lot of time doing something other than practicing math facts.

Solution 2A: Circulate and watch students while they are using the Online Tutor

If possible, monitor students closely while they are doing the Rocket Math Online Tutor.  Walk around, look over their shoulder and see them answer a couple of problems.  Pat them on the back, literally or figuratively, and move on. Praise people who are working hard, and do it aloud so other can here you.  Comment on anything you see, so they know you are watching and know you care how they are doing. Keep moving around the room so you can see everyone.  Keep circulating so that they know you will be coming by frequently.  If you do that you won’t have anyone off task.  If you did this for a month or two, you would build in good work habits and then you wouldn’t need to do it all the time.  If you never do this, you have no idea what is going on in the back corners of your room!

Solution 2B: Post names of those answering the most problems

If you are teaching something else while students are working, you won’t be able to monitor them while they are working.  However, at the end of the day you can go to the Review Progress page.  Scroll down to the individual student rows and scroll over to the Problems Answered Today column.  At the top you can barely see a little gray arrow and a stack of tiles.  Click it once and it sorts from the lowest up to the highest.  Click it again and sort it from the highest down as you can see in this example here.  Then you can post the names of the students who answered the most problems.

Just before students begin working on Rocket Math the next day stand up and read aloud the names and praise those students who are really working hard.  “I know these students are working hard because of how many problems they were able to answer yesterday.  They are going to get really smart and know their facts.  Can you do better?  I’ll see tonight who can do more than 100 problems!  Maybe your name will go on the board tomorrow!”

And of course, you should have a talk with anyone who is doing very little.  They could always do an extra session of Rocket Math during recess or after school if they can’t get more done during the regular time.

 

Still not progressing?  See Part 2

If your student is working hard and completing 5 to 10 sessions a week, they will most likely be making good progress and passing several levels each week.  If they are working hard and still not making progress go on to Part 2 of this blog.

 

 

Student not progressing in Rocket Math Online Tutor? How to diagnose the problem. Part Two

Posted on by Dr. Don

If you have not, please read and implement Part One of this blog post.  You have seen that your student is completing 5 to 10 sessions a week and is working hard the whole time (answering a hundred or more problems each session).  But still the student isn’t making good progress passing several levels a week?  Let’s look deeper into the problems.

Find out if it is too difficult for this student

When students make three errors or three hesitations in a part or a phase of Rocket Math, we have them start over and repeat those problems. In the Review Progress tab, in the individual student rows is a column titled “Difficulty.”  The Online Tutor calculates the difficulty score  by dividing the number of times the student had to “start over” or repeat a part by the number of parts passed.  We expect that students will usually have to start over once or twice in a part, earning them a difficulty score of “1 or 2”  However, if they have to start over an average of 3 times per part, (a difficulty score over 3.0) then it may be too difficult for this student.

In the Review Progress tab, you can look up the difficulty score for any student.  Or as was done in the picture here, you can sort the difficulty column to see the students with the highest difficulty scores at the top.  Then you should watch the students who are having high difficulty scores while they are working to see what the problem is.

Problem 3: Problems using the keyboard quickly

Some students, especially those under 8 years old, are not as adept at using a computer keyboard as they need to be for the Online Tutor.  At the standard speed, answers must be entered within 3 seconds for a one-digit answer with only an additional second for subsequent digits. Some students cannot use two hands so they can quickly hit the answer and the enter key. Searching for and tapping separately with one hand both the numerical keys and the enter key will be too slow–they will have a lot of start-overs. If you watch such a student work, you will see that they know the right answer, but cannot get it input on the keyboard in time.  This will frustrate them and slow down progress.

Solution 3A: Have the student use a touch screen

Using a touch screen is much easier than a computer keyboard.  For children under 8 years old, or students who are having difficulty entering the answers they know getting them a touch screen device should solve the problem.  To test if this will solve the problem, put the free Rocket Math app on your phone, log in for the student and let them do a session on your phone.  If they do better (have fewer start-overs) then you know how to solve the problem.  Touch screens are becoming more common, so we’re hoping you can locate one for the student who is having trouble.

Solution 3B: Slow the Online Tutor answering speed

If a touch screen option doesn’t help–the student is still frustrated then you can adjust the speed required by the Online Tutor.  This will help an individual student who is having trouble keyboarding the answer quickly enough.  Here are the directions (and caveats) about changing the speed of answering. https://www.rocketmath.com/online-tutor-knowledge-base/b-too-hard-or-too-fast-check-the-difficulty-score/

Problem 4: Student is not ready for the Learning Track

Students who are not ready for the Learning Tracks will have a lot of difficulty progressing through the Online Tutor.  Students won’t succeed who have not learned the basic prerequisites, such as understanding the operation they are trying to memorize.  For example kindergarten students who don’t know how to add two groups of objects (by counting) aren’t ready for LT 1-Addition.  Before students understand addition and subtraction they should not be memorizing the facts.  Generally we want first and second graders in Learning Track 1-Addition followed by LT 2-Subtraction.  The same thing goes for multiplication.  Students need to understand what multiplication means and be able to “figure it out” before being asked to do LT 7-Multiplication. After this concept is learned in third or fourth grade is a good time to begin LT 7-Multiplication followed by LT 8-Division.

Solution 4: Stop Online Tutor and teach more basic math skills first

Students younger than these guidelines or who are cognitively delayed below these mental ages may not be successful with the Online Tutor learning tracks. If they aren’t successful, you’ll need for them to develop more basic math skills before using the Online Tutor.

 

 

 

 

 

 

Online Tutor should be assigned as homework–here’s how

Posted on by Dr. Don

We call our app the Online Tutor for a reason.  It patiently teaches like a top-notch tutor would.  Why waste this resource when it can be assigned as a very valuable and easy-to-check-on homework assignment.  You don’t have papers for the dog to eat, or papers to correct, but students will greatly benefit from more time spent each day practicing math facts.  You have already paid for it, so make the most of this resource.

Three ways to access the Rocket Math Online Tutor at home

Of course, the first way is to log into a web browser such as Google Chrome, Firefox, Microsoft Edge (anything other than Apple’s Safari which is not compatible with our app) and go to https://play.rocketmath.com .   You can play from there, but if the student is using a mobile device the apps work better and are free.

Rocket Math has a mobile app that parents can download for free.  The apps can run on a phone as well as other mobile devices, so it is not necessary that children have a computer available at home.  On the Enter page there are two buttons to access the mobile apps.  We have two versions:

one on the Apple App store https://apps.apple.com/us/app/rocket-math-online-tutor/id1538196379  and

one on Google Play https://play.google.com/store/apps/details?id=com.rocketmath&pli=1

You can send these links home so that students can work with the Online Tutor on any mobile device.

Use the Parent Letter to send home login credentials.

You can find the Parent letter on tab (i) Parent Letter on the rainbow navigation bar of the admin account or here.  Print enough copies for your whole class.  Enter the student’s name at the top and the Username, Passcode, and your Account number on the lines provided. (If it were me, I’d then copy them all to have another set for lost copies).  Then you can send these home with students so parents know how students can logon and do a 10-minute session.  The letter explains about the “Session Completed” screen so that students can be encouraged to show that to their parents each evening to prove they have “done their homework.”

On the Parent Letter shows that on the enter page (play.rocketmath.com) parents can access the mobile apps which they can download for free onto their phone or their child’s mobile device.

Promote homework during Back-to-school night.

It will take a little effort to build in this expectation, but it will be well worth it.  Working with the Online Tutor, students can’t help but develop math fact fluency and they never practice errors.  You don’t have papers to grade, so this will be the best homework ever.  There is nothing other than reading fluency that is more important to future academic success than math fact fluency.  Why not add some additional practice as homework?

On Back-to-school night, bring in a couple of students to demonstrate a session for parents, so they can see how it works to correct errors.  You can have parents access the links to download the app right onto their phones and give them the Parent Letter.  (See why I would make copies?)  If during conferences, you can download the app onto their phone and have their child login and use the app right then and there.  Seeing is believing, and parents will see that their children can learn their math facts by using this app.

How do you know which students do Rocket Math as homework?

We’ve got that handled for you.  Simply go to the blue “Enable Daily Progress Report” button on the Review Progress tab on your admin site.   Click on that button and you’ll get a pop-up asking you to verify the email to which the reports should be sent and expecting you to hit the green “Enable” button to get these started.

The Daily Progress Report tells you how many sessions students started and how many they completed yesterday.  So if you had students do a session in school (And you made sure they completed it, right?) they would have a “1” by sessions completed.  If they went home and completed another session they would have a “2” by their name.  So you can tell who did homework and who didn’t, right on this report.  It also tells you who passed which levels. (And therefore earned the right to color those levels in on their Rocket chart.)

How can you motivate your students to do the Online Tutor as homework?

First, you should assign it.  Put it on the board.  Send home notes.  Send home the parent letters–again. (Aren’t you glad you made copies?)  You need to convince your students that this is important and that you expect them to do it.  That’s the start.

But then you need to show them you are impressed by the students who do it as homework.  You may have to read from the daily progress report with disappointment, that nobody did it as homework yet.  But then one day, you’ll have someone who does it as homework.  Have them stand up and give a cheer and get a round of applause.  The next day, do it again. And the day after that.  Eventually you’ll have a few doing it.  Put their names on the board as math superstars.  Keep praising them.  Make up a note to send home with the reminder to do Rocket Math as homework, praising the ones who have been doing it.  Keep acting like you care and are impressed by the ones who are “doing their homework” and eventually almost all of your students will be doing it at home regularly.  Their progress will take off and they will become math success stories.  It’s a lot of work, but it is your mission, right?

 

The Wall Chart motivates and prevents unhealthy competition.

Posted on by Dr. Don

The Rocket Chart gives students a visible sign of their success.

Students working in Rocket Math begin to see themselves as clearly successful.  Being able to color in the levels of their Rocket Chart as they pass them, makes their progress visible.  As they color in the Rocket Chart they become very invested in their progress through the levels. They are naturally proud of their accomplishments. Whenever Dr. Don visits a classroom, students want to tell him what level they are “on” if they get a chance.

Competition may develop to unhealthy levels

However, not all students progress really quickly or easily, while others surge ahead. Sometimes, unhealthy competition may develop among students sometimes.  Some students begin to feel really bad about their slower progress. Worse yet, some students in the lead may begin to act arrogantly or disrespectfully.  The Rocket Math Wall Chart is designed to curb that competition and to build a sense of esprit de corps.

The Wall Chart puts all the students on the same team.

star_sticker

Over 700 star stickers come with the Wall Chart.  Each time a student passes a level the teacher awards them with a star sticker, which they take up to the Wall Chart and put into one of the squares in the chart.  Students fill the chart from the bottom up.  The teacher sets a goal in a few weeks, which date is marked on the goal arrow, and the goal arrow is placed a couple of rows up from where the students are now.  (You can just see that in the picture above.)

 

Students develop pride in their whole class.

If the students fill in the squares up to the arrow–before the date specified on the arrow–they earn a group reward such as extra recess time, or music during math, or a congratulatory note home, or a popcorn party, etc.  Wall chart half filledIn this way, each time a student passes a level they are putting up a score for the whole team.  It is good for everyone.  The teacher is able to praise the class for their hard work and accomplishments, and the whole class is able to feel good about their collective effort.

The Wall Chart shows visitors (like principals) how well the class is doing.

Passers-by as well as interested administrators can praise the class as a whole for their successes with Rocket Math.  A savvy administrator may reward the class with a “free space” to help themselves keep track of progress. See this blog.  In many schools, classes post their completed Rocket Chart on their door with all 725 stickers in place!   The Rocket Math Wall Chart becomes a focus of pride and recognition for the whole class.  The Rocket Math Wall Chart (#2005) includes directions, plenty of star stickers, four goal arrows, and the chart itself.   They are cheaper by the dozen if you need more.

How to Grade 1-Minute Math Fluency Practice Tests

Posted on by Dr. Don

Katy L from Wilson Elementary asks: How can I keep up with everyday Rocket Math grading? Do you teach students to grade their own 1-minute math fluency practice tests?

Dr. Don answers:

Only grade 1-minute math fluency practice tests if students pass

An integral part of the Rocket Math Worksheet Program is the 1-minute math fluency practice test. One-minute fluency practice tests are administered every day, to the whole class, and only after students practice in pairs for two to three minutes each. Check out the FAQs page to learn more about conducting 1-minute math fluency practice tests in class.

Teachers do NOT need to grade, score, or check daily Rocket Math 1-minute math fluency practice tests unless the student has met their goal. Students do NOT need to grade their own daily Rocket Math fact fluency tests either.

Why grading each math test is not important

The important part of math fluency practice is the oral practice with the partner before the test–what’s going on in this picture. Because the students are orally practicing every day and getting corrections from their partners, there should be VERY FEW errors on the 1-minute math fluency written tests.  

Correcting written tests doesn’t help students learn anyway. Corrections are only helpful if they are immediate, the student has to acknowledge the correct answer, and remember it for a few seconds–all of which is part of the oral correction procedure. “Correcting” what’s on the paper takes a lot of time and does not help students learn more, so it shouldn’t be done. But you have to check them before declaring that the student has passed a level.

How do you know if a student passes?

Students should have a packet of 6 sheets math fact fluency sheets at their level. Each Rocket Math student has an individual goal. For example, if a student has a goal of 32 (based on their Writing Speed Test) and they only do 31, they know they did not pass. If the student does 32 or more, they pass!

What to do when a student beats their goal (passes)

If a student meets or beats their goal, then have them stand up, take a bow, and then turn their folder into a place where you check to see that all problems were answered correctly. When YOU check (after school?), make sure all of the completed problems were correct and the student met their goal. If so, then you put the unused sheets in that packet back into the filing crate and re-fill the student’s folder with a packet of 6 worksheets at the next level and hand the folder back the next day.

When students receive the new packet of worksheets, they know to color in another letter on the Rocket Chart (and maybe put a star on the Wall Chart).

What to do if a student doesn’t pass?

Students who don’t meet their goals, don’t pass. These students should put the non-passing sheet into their backpacks and take the sheet home for more practice.

The next day they will use the next sheet in their packet of 6. If you want to give them points, do that the next day after they bring back their worksheet where they did a session at home (signature of helper should be there) and all items on the test are completed. If that’s done, they get full points.

Sometimes you’ll catch errors on sheets that students turn in as “passes.” If you see an error, the student doesn’t pass. As a result, the student keeps the old packet and has to continue with that same level worksheet.

For more information about conducting 1-minute math fluency practice tests in class and how to implement the Rocket Math worksheet program, visit the FAQs page.

Students work with together to learn how to write their numbers.

The 100-point observation form: How well do you implement Rocket Math?

Posted on by Dr. Don

Use the 100-point observation form to evaluate your implementation.

Use the 100-point Rocket Math Observation form to self-evaluate, or have someone observe your class doing Rocket Math and use the form to evaluate you.  The form observes and evaluates seventeen different indicators of the quality of your Rocket Math implementation.

You or your observer begin by looking at four important indicators of the quality of student practice.  The quality of the paired practice of your students provides most of the value of Rocket Math.  Accordingly, these four indicators provide nearly half of the 100 points.  If one or two of these things are not in place (tutors aren’t listening carefully and correcting errors AND hesitations, for example) the implementation will not earn high marks, because students won’t be learning nearly as well as they should or could.

The other thirteen indicators are mostly about the efficiency with which Rocket Math runs.  If it takes more than 15 minutes a day to complete Rocket Math, it won’t happen every day.  If Rocket Math doesn’t happen every day, students do not learn nearly as well as they should or could.

Where can you find the 100-point Observation form?

There are three places you can find this handy form.  (1) It is included in hard copy form in the Administrator and Coach Handbook which we sell and ship to you.

(2) The 100-point Observation form is also available for free on the Resources/Educator’s Resource page on our website where you can find this link to its pdf.

(3) And finally in the Rocket Math subscription filing cabinet, in the Forms and Information drawer, there is a section (pictured on the left) that is devoted to all the information in the Administrator and Coach Handbook and near the bottom you’ll see the 100-point Observation form for you to print out.

Why is a gifted student having trouble with Rocket Math?

Posted on by Dr. Don

Question: Hi, Dr. Don! Just had a question recently from a parent of a gifted child whose son is having a lot of difficulty doing Rocket Math! He understands almost everything conceptually in math (in the 99% on national testing) but he is not being successful working with a partner on his math facts. Have you had this problem in other places? I’m not sure if the problem is he really can’t focus on the facts, he’s stubborn and doesn’t like details (big picture thinker), etc. He’s a very social kid so the partnering doesn’t seem to be the problem. I would greatly appreciate any suggestions you might have that I could give this mother. She says that he is fine at home doing his facts with her without a timer. But I don’t like the idea of excusing any student from doing this valuable practice. Thanks for your thoughts. Linda

Answer: I’ve blogged a bit on some of these issues elsewhere on the Rocket Math website, but let me try to be more specific here. First, gifted kids are stunned to find out that they have to work hard to memorize math facts. They probably need three or four days of practice—which to them seems like failure.  They are like an athletic kid who excels easily at every sport but finds he needs to work out with weights as much as a klutz to get to be able to lift heavy weights—his natural talent doesn’t help in this instance. So kids who’ve never had to work to learn things before, really are annoyed by having to practice several days in a row.  But it is really good for them!

How is mom practicing with him at home? Can she video him doing the test “untimed?”  If the child is “writing facts” and “without a timer” then he may be figuring out facts over and over—but is not getting to instant recall. That’s why the oral peer practice is so critical—if there is even a slight hesitation the child is to repeat the fact three times, back up three problems and come at it again—until the answer comes with no hesitation. There is a fundamental difference between instant recall of facts from memory and strategies to come to the answer by thinking it through. My parent letter addresses how to practice.  On the other hand, if the student is able to write the answers to math facts at a fast enough rate to complete 40 problems in a minute, but only when he thinks he is not being “timed” then he needs to learn how to do the same thing when he is being timed.

If he is not learning with the daily practice, we have to ask, “Why not?”  Social kids sometimes socialize instead of practicing. Social kids also can convince their partner not to do the correction procedure. Or they just say the answers instead of the whole problem and the answer. Any of those things would result in not successfully learning the facts. The teacher would need to monitor the quality of the practice. My experience has been that when students are “stuck” or “having difficulty” even just one session of practice done the right way rigorously (with me) and they suddenly improve enough to pass or to recognize they can pass the next day with another session of rigorous practice.

Last of all, sometimes the writing goals are off because of some glitch in how you gave the writing speed test.  So the student might know the facts well enough but not be able to write them fast enough to pass the tests.  If the student can answer 40 facts in a minute in the current set (just saying the answers without having to say the problems) then the facts are learned to automaticity—and the goal in writing should be lowered to whatever the student has done to this point.

Hope this helps. You are right not to excuse this student from learning math facts to automaticity. He might be a stellar mathematician someday if he learns his facts well enough that math computation is always easy for him. If math computation remains slow or laborious he won’t like it enough to pursue it as a career.

Without the directions you may get lost!

Posted on by Dr. Don

What happens when teachers don’t have a copy of the Rocket Math Teacher Directions?  Bad things!  

When teachers don’t have the written directions to Rocket Math, the essence of the program usually gets lost.  Procedures get modified and modified over the years until they are not even close to what should be occurring. Sometimes we have found schools that are not even providing daily oral practice.  Other schools don’t give the answer keys to the peer tutors.  Other schools don’t give the writing speed test and make up impossible-to-reach goals for students.  We often see teachers implementing the “Rocket Math” program incorrectly and wondering why it doesn’t work.  We ask them if they have read the teacher directions, and they say they didn’t know there were any.  When teachers have never seen the directions, is it any wonder they don’t know what they are supposed to be doing?  Hear-say directions handed down over the years from one teacher to another just don’t convey all the important details.  Teachers need the directions!

This is why I’d like you to have my complete directions for free. Even if you purchased Rocket Math ten years ago and haven’t gotten the updated versions since then, you can have these directions for free.  I have them in three places.  I have the directions broken out into FAQs on their own web page here.  That’s easy for quick reference.

The second place I have the Teacher Directions is as a downloadable booklet you can print out and distribute.  The Rocket Math Teacher Directions for the worksheet program booklet is here.   Please print this out and give to your teachers, especially in schools that began implementing several years back.  Read them and have a discussion at a professional development time.  You will be astounded at how much your implementation differs.

The third place I have the Teacher Directions is in the “filing cabinet on the web” for those of you who have the subscription. In the “Forms and Information” drawer we have the booklet and the FAQs which can be opened and printed out.

In school-wide implementations of Rocket Math, principals or math coaches need to take a leadership role.  The Administrator and Coach Handbook gives you forms with what to “look-for” in a Rocket Math implementation.  If you use that to observe Rocket Math in your classrooms you’ll quickly see whether or not things are going the way they should.   If you have a subscription to Rocket Math you’ll find all of the chapters of the Administrator and Coach Handbook in the “Forms and Information” drawer of our filing cabinet on the web.

Please take the time to see that you or your teachers are implementing Rocket Math according to the directions.  Trust me, it works SO MUCH BETTER if you do.  I wouldn’t steer you wrong!

 

Rush help to those who need it with an aimline

Posted on by Dr. Don

The sooner you provide extra help the easier it will be to catch them up.  

How can you know when students need help to meet expectations?  Use the graph above, which is available from the Educator’s Resources page or here: One Semester Aimline.  It is also available in the basic subscription site, Forms and Information Drawer as an optional form. It is an “aimline” for finishing an operation (Sets A-Z) in one semester.  Schools that don’t start Rocket Math in first grade need students to finish addition in the first semester of 2nd grade and subtraction in the second semester.  This means that students who get stuck on a level for even a week need to be helped.

If you indicate on this graph the week in which the student finishes each set in Rocket Math you can tell if the student is making enough progress, or if he/she needs to be getting extra practice sessions each day. If the student is working on a set above the line of gray boxes or on the line then progress is adequate–they are on track to finish the operation by the end of 18 weeks of the semester.  But if the student is working on a set that is below the line that means he/she needs intervention.

In the example above the student whose progress is shown in red is above the aimline.  That student has been passing at a rate that means he or she will finish the operation by completing Level Z by the end of the semester.  That student does not need any extra intervention.  In the example above the student in blue is falling behind.  By the fourth week that student has only passed Level C and so he needs to have extra help.

The first step would be to ensure this student has a good partner and is practicing the right way.  Sometimes students don’t stay on task or do not listen and correct their partner.  If hesitations are allowed (while the student figures out the answer) and not corrected the student will not improve.  Fix the practice in class first and see if the rate of passing improves and the student starts to get up to the aimline.

The second step is to include this student in a group of students who get a second practice session each day.  They would work in pairs and do another Rocket Math session each day.  Whether or not they take tests is unimportant.  What is important is that they do the oral practice with a partner who corrects their hesitations as well as their errors.  This could be done by a Title One teacher or assistant or a special education teacher or assistant.  It should only take ten minutes.

Another step is to involve parents if that’s possible.  Another practice session (or two) at home each evening would make a big difference.  Parents will need to know how to correct hesitations, but there’s a parent letter in the Forms and Information drawer for that.  Also note that siblings can do this practice as well, as long as they have an answer key.

You will be pleasantly surprised at how an extra few minutes a day of good quality practice can help students progress much faster at Rocket Math.  The sooner you intervene, the easier it will be for the student to catch up.

NOTE: There is an aimline for finishing one operation in a year.  It is also in the Forms and Information drawer and on the Educator’s Resources page of our website.  If you follow recommendations and do addition in first grade, subtraction in second, and multiplication in third you can use that aimline.  It won’t require intervening on so many students.

 

 

What about students who can’t pass in 6 tries?

Posted on by Dr. Don

A teacher writes:

Help! I’m feeling bogged down in Rocket Math. I have some students who have been working on the same sheet for over 10 times and are no closer to passing. What am I doing wrong?

Dr. Don answers:

The problem could be one of several things.  You have to diagnose what it could be.  I am assuming you have students practicing orally in pairs, with answer keys, for at least two minutes per partner every day (as shown in the picture above).  I am assuming you already have students, who do not pass, take home the sheet on which they didn’t pass and finish it as homework and practice with someone at home.  The extra practice session at home each day can be a big help and the students should be motivated to do that.   If this is the case and you still have a problem, below are two possible things that may be needed.

(#1) Need to improve practicing procedures.  Pick one of the students who is stuck and be that student’s partner while they practice orally.  Make sure they are saying the whole problem and the answer aloud so you can hear what they are saying.  Correct even any hesitations, not just errors.  Correct the student by saying the correct problem and answer, having them repeat the correct problem and the answer three times, then back up three problems and move forward again.

Diagnosis.  If, after practicing with you, the student does much better on the one minute timing and passes or nearly passes (this is what I usually found) then you know the problem is poor practicing procedures.  If your work with the student makes no difference (they don’t do better on the one-minute timing) and they seem equally slow on all the problems then it is not practicing procedures at fault.  Try #2

Solution:  Monitor your students closely during oral practice to see if they are all following the correct practice procedures.  If you have quite a few students who aren’t practicing well you may need to re-teach your class how to practice.  [Note: Even if they know how to do it but aren’t doing it right, treat it as if they just don’t know how to to do it correctly.]  Stop them and re-do the modeling of how to practice and how to correct for several days before allowing them to practice again.  If your students haven’t been practicing the right way, they won’t be passing frequently, and they will be unmotivated.  You have to get them practicing the right way so they can be successful and so they can be motivated by their success.

Solution:  If you have poor practicing with only a handful of students you might assign them to more responsible partners and explain to them that they need to practice correctly. During oral practice monitor them more carefully the next few days to be sure they are practicing better and passing more frequently.

(#2) Need to review test problems also.  The problems practiced around the outside are the recently introduced facts.  The problems inside the test box are an even mix of all the problems taught so far.  If there has been a break for a week or more, or if the student has been stuck for a couple of weeks, the student may have forgotten some of the facts from earlier and may need a review of the test problems.

Diagnosis.  Have the student practice orally on the test problems inside the box with you.  If the student hesitates on several of the problems that aren’t on the outside practice, then the student needs to review the test items.

Solution. If you have this problem with quite a few students (for example after Christmas break) then have the whole class do this solution.  For the next three or four days, after practicing around the outside, instead of taking the 1 minute test in writing, have students practice the test problems orally with each other.  Use the same procedures as during the practice—two minutes with answer keys for the test, saying the problem and the answer aloud, correction procedures for hesitations, correct by saying the problem and answer three times, then going back—then switch roles.   Do this for three or four days and then give the one-minute test.   Just about everyone should pass at that point.

Solution.  If you have this problem with a handful of students, find a time during the day for them to practice the test problems orally in pairs.  If the practice occurs before doing Rocket Math so much the better, but it will work if done after as well.  They should keep doing this until they pass a couple of levels within six days.

If neither the first or the second solutions seem to work, write to me again and I’ll give you some more ideas.