teaching

Improving math achievement: what’s unique about Rocket Math?

Posted on by Dr. Don

Rocket Math is dedicated to improving math achievement. Long-time educator Dr. Don Crawford founded Rocket Math because of his passion for effective educational tools. He believes all students can succeed in math and dedicated his company to making it happen. Their achievement and success motivate students more than anything else. Rocket Math’s mission is to help students succeed in math.

Motivated by success.

Rocket Math’s Online Game educational app teaches and develops fluency in basic math facts. The app is unique in a couple of respects. First, the game focuses students on their progress in learning math facts rather than distracting them with a cutesy game format. As students fill in their individual Rocket Chart, they become motivated by their progress in learning the facts and developing fluency. Moreover, they also develop confidence and improved self-esteem as a by-product of the process.

Evidence of effectiveness in teaching.

Second, the rocketmath.com website uniquely provides real-time evidence of its effectiveness. Rocket Math charts all its users, showing they are learning and developing fluency with basic math facts. Go to their Evidence of Effectiveness page, and you can see students’ results on fluency tests. The tests are given four times in each learning track (pre-test, 1/3 through, 2/3 through, and post-test). For example, over 55,000 students have completed the Multiplication learning track. The chart shows they began with an average fluency of 11 problems per minute at the pre-test. Those students finished the Multiplication Learning Track with an average of 21 problems per minute. Student scores show they are more fluent at each milestone in all 16 learning tracks, from beginning Addition to Fraction and Decimal Equivalents.

The most powerful thing you can do to improve math achievement.

Helping students develop fluency with basic math facts is the single, most powerful thing school administrators can do to improve student math achievement. There’s no excuse for allowing students to struggle and count on their fingers or rely on multiplication charts while trying to do math. Rocket Math has the mission of fixing that for anyone who uses their app. They even offer a 30-day initial, complimentary subscription so you can see that it works before paying a dime. Rocket Math has a money-back guarantee that using their app will improve student fluency in math facts. Rocket Math continues to grow thanks to the enthusiasm of its customers.

Things-to-look-for (any time of day) for Rocket Math implementation

Posted on by Dr. Don

Evaluating a Rocket Math implementation when you aren’t observing Rocket Math in action.

Most of the time when you go into classrooms, something other than Rocket Math® will be going on. These are the things you can check on even when there are no students in the room. There are eight indicators you can see by looking at student folders.  There are four indicators while looking at the Rocket Math filing crate.  There are additional indicators to look for if there is a Wall Chart being used or if there are Race for the Stars games in the room.  Here’s a link to the checklist.

Look at several Student Folders

(1). Students all have folders that appear to be used daily. The folders are the heart of the organizational system. Students should keep their materials in the folders and keep track of their progress on the folders. Whether the students keep folders in their desks, cubbies, or are collected each day, there should be some signs of wear and tear.

(2). Rocket Charts on student folders show dates of each attempt to pass a level. Each day when students take a 1-minute timing test to try to pass a set of facts, they should write the date of the “try” on the Rocket Chart on the front of their folder. Without this record you cannot tell if a student is stuck because he or she has missed two weeks of school, or if students are only doing Rocket Math® twice a week (not recommended!), or if a student has exceeded six tries without intervention.

(3). Rocket Charts on student folders are colored in when passed. Coloring in the row on the Rocket Chart for the fact set that was just passed is the primary reinforcer of all that hard work. It is essential that students are given the time (and the colored pens, pencils, or crayons) to celebrate their success. Don’t get fooled by the older students or the students who are “too cool” to color in the chart. Even if they only want to color in the row with their regular pencil, students need to be told that they have accomplished something important, and giving them the time to color in their chart is a critical component of the program. This is way more important than you might think. You can also praise students who have accomplished a lot or who have just passed a level. Hearing from an administrator or coach about progress in math facts sends a huge message regarding the importance of the task.

(4). Student folders include packets of answer keys on colored paper. In order to practice correctly, each student’s partner needs to have an answer key in front of them when practicing. Each student needs their own answer key packet (so they can practice with someone who doesn’t have that answer key or with a volunteer who has no answer key). All the answer sheets for their operation should be copied and stapled into a booklet so students don’t have to go hunting for answer keys. Having the answer keys copied onto a distinctive color is important for teachers to be able to monitor paired practice. When students are practicing, each pair should have one student with answers (in that distinctive color) and the partner without the answers (on white paper). Any variation of this means the students are not practicing correctly—and that should be easy for the teacher to spot. Additionally, if a teacher is ready to begin testing and sees a hot pink paper on a desk, the teacher knows someone has answers in front of him or her.

(5) Student folders have the next sheet ready before starting practice time. Some system needs to be put in place so that the limited amount of time available for students to practice is NOT taken up with all students trooping up to the crate to get the next practice sheet each day. The recommended system in the Teacher Directions is to refill student folders when they pass a level, after school, with a packet of six sheets. That way the only time teachers have to handle folders is when students pass and they check the “pass” for errors and refill with a new packet. Many other ways of refilling student folders are possible, but no matter the process, students should have a blank practice sheet or set of practice sheets in their folder—which you would see when you check folders.

(6). Students have clear goals indicated on goal sheet. After students complete the Writing Speed Test, they are to have goals set for their daily 1-minute timing. The goal sheet should be stapled to the inside left of the student folder, the goal line circled, and the 1-minute goal written at the bottom of the sheet. The goal may be crossed out and a higher goal written in if the student has consistently demonstrated the ability to write faster than the original goal. Sometimes, teachers also write the goal on the front on the Rocket Chart, but the student’s goal should be clearly indicated. If not, it may be arbitrary or inappropriate (the same for all students, for example).

(7). Individual graphs are filled in because 2-minute timings are happening. Every week or two, students should be taking the 2-minute timings. These timings are a progress monitoring measure. They could be used for RTI or for IEP goals, or for any other time when a curriculum-based measurement is useful. At least they can demonstrate to us (and to the students) whether they are making progress in learning math facts in a given operation. As students learn more and more facts in the operation to a level of fluency and automaticity, they will be able to write answers to more facts in the operation on the 2-minute timing. Each time they take a 2-minute test, they should count the number correct and graph that on the graph stapled on the inside right of their folder. Each test is graphed in the correct column for whichever week of the month the test was taken.

(8). Individual graphs show upward trends as students are learning facts. Once students are taking the 2-minute timings regularly, it should be easy to see a trend. It should be going up, even if somewhat unevenly. For example, scores might go down after the long December break, but they should recover after a couple of weeks. If these graphs do NOT show an upward trend, something is wrong. Practice may not be being done for long enough (less than 2 minutes a day), or frequently enough (only three times a week), or students may not be practicing correctly (not fixing hesitations and errors). If only one or two students have flat graphs, those students will need something more. The individual graphs will be your indication that there is something amiss. You will just have to figure out what could be wrong. This should lead you to do some observations during Rocket Math® practice in that classroom.

Look at the Rocket Math file crate

(1). There is a crate or set of files for each operation practiced in the room. Each operation fills a crate and requires a different set of files. In any classroom where not all students are working on the same operation, there will need to be more than one set of files. Sometimes, teachers who have only one or two students in an operation may use the files of a neighbor teacher, but that should be only a temporary fix. The rule is that there must be a crate for every operation being practiced in that class.

(2). Rocket Math® crate is filled and organized from A–Z, complete with tabs. As of the 2013 version of Rocket Math®, every operation goes up to the letter Z. So each crate should have hanging folders with tabs showing the letters A though Z. Tabs are important to save time finding sheets and filling folders. If the files are a mess, out of order, no labels, or some letters are empty, valuable practice time will be used up trying to find the right sheets. If everything is labeled, and there are sheets in each file, then efficiency is a possibility. The Rocket Math store has tabs for sale if you need them.

(3). Rocket Math® crate has 2-minute timings numbered 1–5. In order to make sure that teachers do the 2-minute timing and monitor progress readily, they need to have class sets of the 2-minute timings (1 through 5) available in the crate. This is easy for you to check. If they are not there, it is likely that the 2-minute timings won’t be done as regularly as they should be. It is important for those timings to be done so you can see if all the students are making good progress.

(4). Teacher has a hard copy of the directions available for reference. The best place to keep the directions is right in the crate, so they are handy at any time. We have found that most of the time, when teachers are not doing things as they should in their Rocket Math® implementations, they don’t have a copy of the directions. When teachers don’t have the directions handy, they will ask a colleague how to do things. Unfortunately, this is like a game of telephone and typically doesn’t end well. Being sure that every teacher has the directions available for easy reference goes a long way toward proper implementation. It also allows you to pick up the directions when you are in the room and point something out to the teacher or to reference an appropriate page number in the directions in your notes to the teacher.

You can print the Teacher Directions from the virtual filing cabinet, in the Forms and Information drawer, under Rocket Math Teacher Directions.  You can buy printed copies from RocketMath.com/shop.  There are additional things to look for on the form but they are optional and go with supplemental parts of the curriculum.

How best to do peer teaching?

Posted on by Dr. Don

Why use peer teaching?

Compared to one teacher talking and a classful of students listening, peer teaching can greatly increase student engagement, and can massively increase time-on-task. Listening to a room full of students working together, practicing, and learning in pairs can be a thing of joy. If it is done right, there is nothing more effective for student learning. Research has shown that not only the student rehearsing but also his partner, the student checking the facts, learns from the process. Because all students can be fully engaged, a lot of practice can be accomplished in a short amount of time. However, sessions have to be structured carefully, and the task has to be something that lends itself to peer teaching.

What tasks lend themselves to peer teaching?

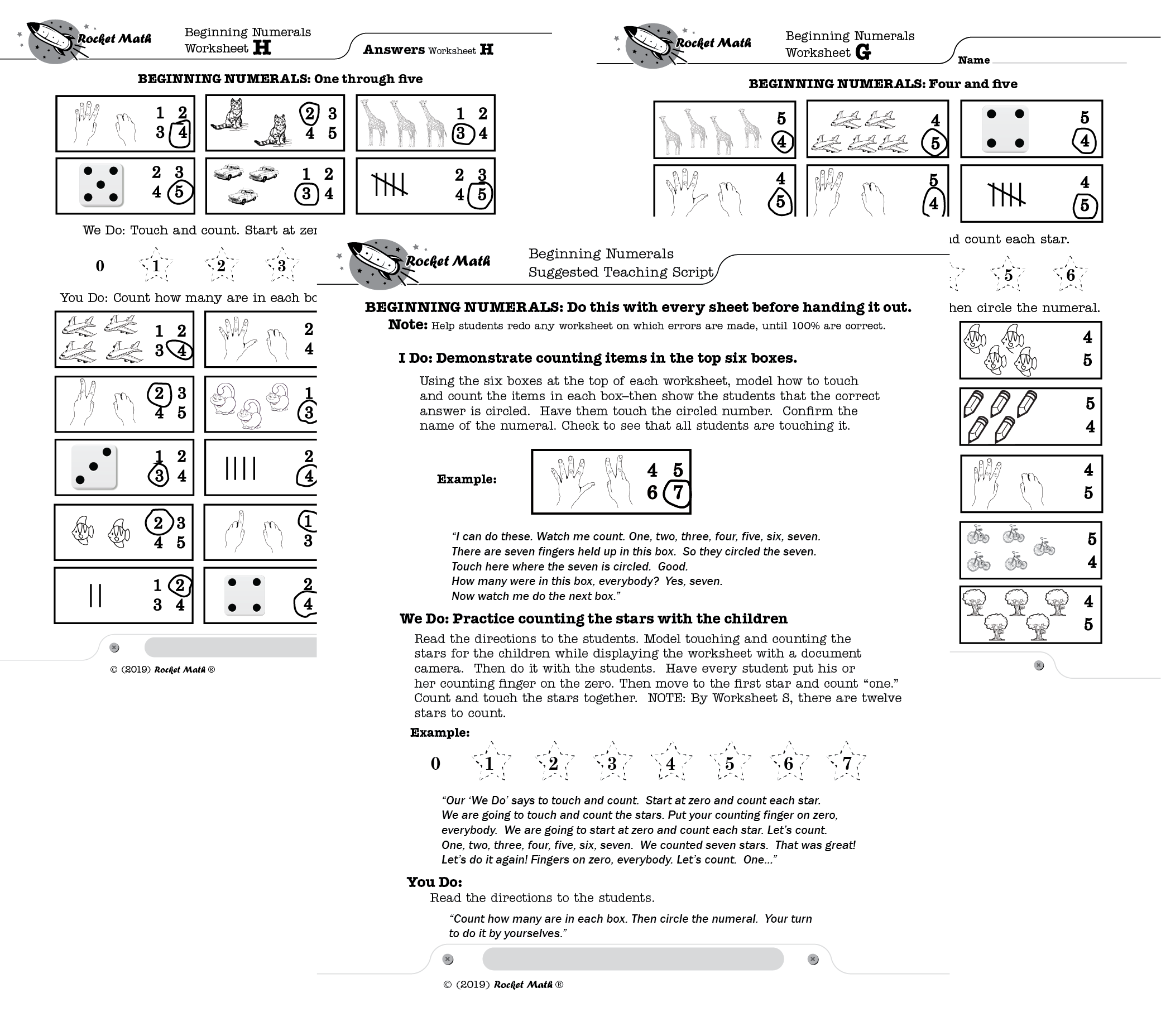
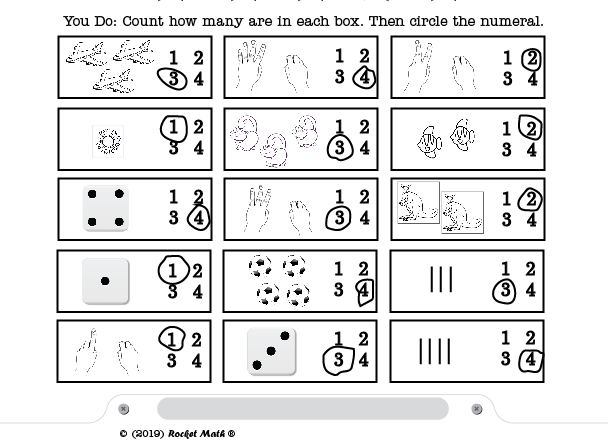
Peer teaching can’t work if neither student knows the material to be learned. You’ll have paired activities, but it won’t enhance or develop learning. Tasks that involve practice and review of previously taught material do lend themselves to peer teaching. Even better are tasks in which one student can have the answer key. You can be sure the correct answers are being learned with an answer key. Being corrected when you make an error is a key to learning, and that is not likely to happen without an answer key. The Rocket Math Worksheet Program is a good example of peer teaching. It involves paired practice of math facts, where one student practices and the other checks on an answer key.

How do you set up peer partners?

If you want to accomplish learning rather than facilitate socializing, you must set up peer partners. There is a saying, “Water seeks its own level.” This is definitely true of student pairs. Left to their own devices, the hard-working, conscientious students will pair up; unfortunately, the goof-offs will also pair up. And they won’t get anything accomplished. If you have an activity where it doesn’t matter what they accomplish, then it’s fine to let students pick their partners. But when you want them to be on-task and learning from the activity, you must set the partners.

Order your class list by focus and responsibility from top to bottom, then divide the list in half. Match the second half with the first half so that top students go with middle students and middle students go with bottom students. (See the picture to the right to get the idea.)

You want to have a responsible, on-task type student in each pair. You can avoid bitter enemies or students who have had problems in the past. But you do not need to match students up with their friends. They are here to practice, not to socialize. Also, do not give in to students who complain about their partners. Tell them “This is going to give you a chance to practice your ‘niceness skills’ which are important to learn. Even if you don’t like them, just do your work and practice your ‘niceness skills.'”

If you do have a volatile situation, you can change the partners, but be sure to change several pairs to obscure the real reason for the change. If students realize they can get out of having a partner by creating a bunch of drama, you’re in for a long year.

How do you avoid a lot of time lost in transition?

Once you’ve set up the partners, you have to set up a routine for “getting with your partner.” You can have a bunch of different solutions for getting with your partner. Some students may just turn around, while others bring a chair, and still others meet at a different part of the classroom. You need to explain to each student in each pair how they will “get with your partner.” 

Then once you have established that, you need to practice several times in row, “getting with your partner.” You want them to move smoothly and quickly, arriving with the correct materials and getting ready to begin immediately. Students must practice this several times, and perhaps a couple of days in a row. You want to stress that this should happen quickly and quietly. This is not a time to catch up with your friends or visit a new part of the classroom. Prompt the students with something like this, “Getting with your partner should happen how, everybody?” Students should answer with, “Quickly and quietly.” Then consider timing the transition to go for a record. You will be amazed at how quickly this can happen if everyone is focused, and a routine has been established. When you have quick and quiet transitions in your room, that’s the mark of a real pro!

How can you ensure effective practice and corrections?

You are going to have to teach an explicit set of procedures to students, so they know how to engage with each other. You will need to explain how to practice as well as how to correct errors. Then after teaching the correction procedure, you will need to make ALL of your students model the correction procedure. You do this by role-playing yourself as a student and calling on students to be your tutor/checker while everyone listens. Then you role-play making errors, so your tutor/checker can model the correction procedure. This lets you know if students are ready to work in pairs because they have demonstrated the correct procedures working with you.  Rocket Math its own script which you can use for how to get your students to model corrections. 

How do you keep the students on-task?

You must make the activity into an “endless task” that can continue until you say stop. That way, everyone must keep working, and there’s no excuse to stop. If there is an acceptable reason to stop working, e.g., “We’re done,” then students will stop working. When students can finish a task, they will. What’s more, they will say they are finished (because you can’t tell) even when they are not. Some pairs may never begin. You want a situation where everyone has to be working all the time, so you can have the same expectation for everyone the whole time. This is the reason students practice facts in Rocket Math in a circle, so they just keep practicing around and around until the teacher says stop. That’s an “endless” task, which is key to keeping students on task.  

You have to actively monitor the whole-time peers are practicing with each other.

Unfortunately, this is not a good time to get the attendance roster turned in. Or catch up on grading. You must treat this as an important activity if you want the students to do the same. You need to circulate among the students the whole time. You’ll need to bend down to get your ear next to their practicing so you can hear what is actually going on. You’ll be looking for student pairs that are following the approved (and modeled) correction procedure. When you hear that, stand up and publicly praise that pair so everyone can hear. “Wow, I just heard Tom and Betty doing a perfect correction procedure. They are really going to learn this material well. They are putting forth a real college effort.” Of course, if students are not on-task, be sure to remind them, and circle back to that pair soon, so they can redeem themselves by getting back on task.

How do you handle student disputes and controversy?

When a pair of students come up with a complaint, you can’t adjudicate it because you weren’t there! Therefore, repeat this mantra, “The checker is always right.” Then every time there is a dispute, repeat your mantra, “The checker is always right.” That means the checker’s ruling decides the issue, and you won’t overrule the checker, no matter how eloquent the complaint. If you keep saying the same thing all the time, like a broken record, students will come to realize you’re just going to say, “The checker is always right.” They will soon stop complaining altogether. Which will be a thing of beauty when it happens.

Peer teaching is only effective if managed well.

As you can see from the foregoing, there are several key management strategies that you need to employ to make peer teaching effective. 

  • You need to have the right kind of task assigned and to provide answer keys. 
  • You must set up the peer partners so that you have at least one conscientious worker in each pair. 
  • You need to establish a routine and speedy transition for students to “get with their partner” for peer teaching to begin. 
  • You need to teach students how to correct errors and ensure they’ve learned the procedure by making them model it.  
  • You must set up the task to be “endless” so that no students can get off-task because they are “done.”
  • You must actively monitor student engagement the whole time they are working. Actively monitoring means walking around, listening to them work, and loudly praising those who are doing it right. 
  • And finally, you have to teach them the mantra, “The checker is always right,” to settle disagreements and controversies. 

If you do this right, it will become your favorite time of the day. I know because it always was for me. 

To learn more teaching strategies to incorporate into your class, read my Teaching Strategie blog posts. From benchmarks to worksheets for kindergarteners, Rocket Math has all the tools to help push your students to success!

 

 

 

 

A mom congratulates her son at a baseball game.

Positive Praise: Building Effective Teaching Habits

Posted on by Dr. Don

Positive praise is one of the most effective ways to encourage wanted behaviors from students. Because building habits is not an easy task, here are a few things you can do to start easily incorporating positive praise in the classroom.

  1. Be prepared with positive phrases
  2. Develop the most effective wording
  3. Start Small with two areas you would like to see improved behavior
  4. Practice in the Classroom and watch the effect it has on your students
  5. Grow and expand your positive phrases over time as you master the habit

Be Prepared with Positive Praise Phrases

I distinctly remember trying to help pre-service teachers build the teaching habit of positive praise. I would make suggestions and then observe. Trying to implement my suggestions wasn’t as easy as you would imagine – these teachers would glance in my direction and start the sentence “I like the way you’re . . .” and then trail off without knowing what to say.

Teachers want to use positivity and affirmation with their students, however, in my experience, they don’t always have the appropriate words ready to praise good behavior. Building the teaching habit of positive praise starts with getting the right words ready.

Recently I was reminded of this key component of building the new habit of making more positive statements. I wanted to personally develop this positive statement habit, but for some reason was not making the progress I had hoped for.

I quickly realized that I was making the same mistake I had watched the pre-service teachers make. I was unable to make more positive statements because I did not have any in mind that were ready-to-use.

To build the habit of making more positive statements, I would have to start memorizing some key phrases to keep on standby, ready to use when I needed them.

Positive Praise Example Phrases: How to Develop the Right Wording

The first step in positive praise is learning and developing the most effective wording. Using effective wording means you are getting through to your student, and clearly communicating that you appreciate the good behavior they are exhibiting.

Praise is most effective when it is prompt – when you deliver the praise in the moment. Can you picture a specific scenario in your classroom when many of the students are not doing as you asked, while a few students are dutifully following instructions?

This is the perfect scenario to use positive praise not only in rewarding students with good behavior but also encouraging other students to follow suit. Don’t be afraid to praise good behavior loud and proud for the rest of the classroom to hear!

Here are some examples of positive praise:

  • Look at Alan so smart sitting in his seat and showing me he is ready to learn. Way to go, Alan.
  • I see Beto is tracking with his finger while Claudio is saying the facts. That’s the way to help your partner!
  • Julia, you are so sharp having your eyes on the teacher, so you can learn!  I am impressed.
  • Stacy and Sophia know just what to do, they have their books open to page XX.  They are so on top of it!
  • Fantastic, Justin! You put your pencil down and are waiting for directions.  I can tell you’re going to college.
  • Stephanie is being such a great on-task student by working quietly and not talking.

Start Small: Pick Two Key Behaviors You Would Like to See More Of

Start out by choosing wanted behaviors from the two most annoying or frustrating scenarios you face as a teacher.  Stating small will help you build a consistent habit of giving positive praise.

Take these two wanted behaviors and build two praise statements you can easily use in-the-moment. Make sure the statement names the behavior specifically. Always include the student’s name, and keep it simple and affirmative.

Now, take a note card or piece of paper and write down these two statements. Don’t wait! Write them down now and keep this note in front of you while you teach. It will serve as a reminder throughout your day to incorporate positive praise as much as possible.

Practice saying these phrases aloud until you have them memorized and can recall them without having to think about it. The most important step in building this habit? Actually practicing positive statements in the classroom.

With these key components and diligent practice in the classroom, you will quickly build the habit of positively praising your students.

Positive Praise in The Classroom: Will it Make a Difference?

Fortunately, positive praise is free and can be implemented at any time throughout the school year. Start using positive praise now, and watch how your students respond.

Prepare yourself for giving positive praise when you are about to begin those frustrating scenarios. When the activity begins, look for opportunities to praise the behavior you are looking for when you notice students who are off-task.

You will see results when you use positive praise genuinely and with enthusiasm. You will know it is working if you watch for those distracted students taking notice of who is being praised. If you notice this happening, keep it up. The more praise you give for wanted behavior, the more that behavior will occur.

Grow and Expand Your Positive Praise Habit

Now that you know how to promote a specific behavior with positive praise, you can systematically develop statements for all your troublesome areas.  Every time students are not doing what you want, think of what you want them to do instead.  Behavior analysts call those replacement behaviors. 

Positive praise can also be used creatively alongside other motivational tools in the classroom. When I began my teaching career I was in the habit of scolding behaviors I did not want. Early in my career, I learned the effectiveness of positive praise and began incorporating it into my daily routine.

When I saw the behavior I wanted I would give loud and proud praise for all to hear. I decided to couple this by adding marbles to a jar every time I gave praise, as an added motivational tool – so students could see how well they have been doing. It worked wonders on increasing wanted behavior.

Building new habits is never easy, but I can personally say that as a teacher, learning to incorporate positive praise into your teaching routine will not only help students learn, but it will save you a lot of frustration!

If you are currently looking for a job as a math teacher abroad, check out this link on Jooble.

Rocket Math Adds Beginning Numerals & Counting Program

Posted on by Dr. Don

A screenshot of Rocket Math’s Beginning Numerals counting worksheet showing how students choose the numeral besides the images to show how many objects are in an image.

Beginning Numerals and Counting

Dr. Don has created another math program and put it into the Universal level virtual filing cabinet at Rocket Math. This is a beginning program for kindergarten students and is to help them learn counting and numerals. That means they can’t learn on their own, the teacher must provide instruction. Teachers can use the counting objects kindergarten worksheets to effectively teach students to count objects aloud and then match the word with the numeral. You can see the top half of Worksheet A above.

If you’re already a Rocket Math Universal Level subscriber, you can find the worksheet in your virtual filing cabinet. Not a subscriber yet? Get the counting worksheets.

I Do: Demonstration of Counting

Each worksheet begins with a demonstration of counting objects and circling the numeral that matches. On Worksheet A, there are only the numerals two and three to learn. The teacher demonstrates (best with a document camera so all students can see) how she counts the objects and then points out that the answer is circled. Suggested teaching language is something like this,

“I can do these. Watch me count the frogs. One, two, three.. There are three frogs in this box. So they circled the three. Everybody, touch here where the three is circled. Good.

How many frogs were in this box, everybody? Yes, three.

Now watch me do the next box.”

 

We Do: Counting Together

In the “We Do” portion of the worksheet, the teacher counts the stars first as a demo and then with the students. Worksheet A you all just count three stars. Suggested teaching language is something like this:

“Our ‘We Do’ says to touch and count. Start at zero and count each star.

We are going to touch and count the stars. Put your counting finger on zero,

everybody. We are going to start at zero and count each star. Let’s count.

One, two, three. We counted three stars. That was great!

Let’s do it again! Fingers on zero, everybody. Let’s count. One…”

By Worksheet S the teacher and the students are counting 12 stars together.

The program has a page of teacher directions with suggested language for teaching the worksheets.

 

You Do: Independent Counting

A screenshot of the worksheet portion You Do, with a grid of three by five squares each with images to count and numbers to choose from.

In the “You do” portion of the worksheet (after learning the numerals with the teacher), the students are asked to count the items in each box and circle the correct number. They are not asked to form the numerals–that’s numeral writing skill. They just identify the numeral and circle it. Besides cute items, there are also dice to count, fingers to count, and hash marks to count–so students can learn multiple ways of keeping track of numbers.

Passing a level requires 100% accuracy. Students who make any errors should be worked with until they can complete the worksheet independently and get all the items correct.

 

Rocket Math’s Counting objects worksheets for Kindergarten

This Beginning numerals program will build strong beginning math skills for kindergarten students learning the meaning of numerals. Combined with Rocket Writing for Numerals it will set students up for success in elementary math.

If you’re already a Rocket Math Universal Level subscriber, you can find the worksheet in your virtual filing cabinet [use your link]. Not a subscriber yet? Get the counting worksheets.

 

 

Math Teaching Strategy #1: Help students memorize math facts

Posted on by Dr. Don

Once students know the procedure, they should stop counting and memorize!

When it comes to math facts like 9 plus 7 or 8 times 6 there are only two things to know.  1) A procedure to figure it out, which shows that you understand the “concept.”  2) What’s the answer?

It is important for students to understand the concept and to have a reliable procedure to figure out the answer to a math fact.  But there is no need for them to be required to use the laborious counting process over and over and over again!  Really, if you think about it, even though this student is doing his math “work” he is not learning anything. 

Math teaching strategy:  Go ahead and memorize those facts.

(It won’t hurt them a bit.  They’ll like it actually.)

Once students know the procedure for figuring out a basic fact, then they should stop figuring it out and just memorize the answer.  Unlike capitals and countries in the world, math facts are never going to change.  Once you memorize that 9 plus 7 is 16, it’s good for a lifetime.  Memorizing math facts makes doing arithmetic MUCH easier and faster.  Hence our tagline

Rocket Math: Because going fast is more fun!

Memorizing facts is the lowest level of learning.  It’s as easy as it gets.  But memorizing ALL the facts, which are similar, is kind of a long slog.  Some kids just naturally absorb the facts and memorize them.

Math teaching strategy: Find a systematic way for students to memorize.

A lot of students don’t learn the facts and keep counting them out over and over again.  They just need a systematic way of learning the facts.  Students need to spend as much time as necessary on each small set of facts to get them fully mastered.  If the facts are introduced too fast, they start to get confused, and it all breaks down.  Each student should learn at their own pace and learn each set of facts until it is automatic–answered without hesitation and without having to think about it.  This can be accomplished by everyone, if practice is carefully and systematically set up.  It should be done, because the rest of math is either hard or easy depending on knowing those facts.  And don’t get me started about why equivalent fractions are hard!

 

Math teaching strategies #3: Teach computation procedures using consistent language

Posted on by Dr. Don

Improv can be entertaining, but it will frustrate students trying learn a procedure.

Much of math, and especially computation, is about learning a process or a set of procedures. [I am assuming you are practical enough to know that we cannot expect elementary aged children to re-discover all of mathematics on their own, as some people recommend.]

Learning a procedure means knowing “What’s next?”  If you ever learned a procedure (for example a recipe) you know that it is between steps, when you ask yourself, “What’s next?” that you need help from the written recipe.  Students are no different.  Just showing them what to do is usually not enough for them to be able to follow in your footsteps.  You need to teach them the steps of the procedure.  As with anything you teach, you are going to confuse your students if you do things in a different order, or with different words, or different steps.  What you call things, and some of how you explain yourself, and some of the sequence of doing the procedure is arbitrary.  If you are improvising you will do things differently each time and your students will be confused.  At a minimum you need  it written down.

Math teaching strategy: Use a script or a process chart to keep the instructions consistent.

We know a lot about how to help students learn a procedure.  We know we need to consistently follow the same set of steps in the same order, until students have learned it.  We know we need to explicitly tell students the decisions they must make while working so they know what to do and when, in other words, we have to make our thinking process overt.  We know we need to be consistent in our language of instruction so that students benefit from repetition of examples.  And finally, we know we need to careful in our selection of problems so that we demonstrate with appropriate examples how the new process works and where it does not work.

Guess what?  You can’t do all of that when you are improvising your instruction and making up the directions on the fly. To be able to do all that, you need a script and pre-selected examples.  Many teachers have been taught to use a chart of steps, posted in their classroom, to which they refer as they model a procedure.  The same effect can be achieved with a script, so that the teacher uses the same wording along with the same steps in the same order.  If you improvise, it won’t always be the same, which will confuse your students.

You have to learn when and how to make decisions.  Every math procedure involves looking at the situation and making decisions about what and how to do what needs to be done.  You have to know what operation to use, when to borrow, when to carry, where to write each digit and so on.  Because you as the teacher already know how to do the procedure, it is tricky to remember to explain your thinking.

Math teaching strategy: Teach a consistent rule for every decision students must make.

Good teaching involves first explaining your decision-making and then giving your students practice in making the right decision in the given circumstances and finally to make them explain why–using the rule you used in the first place.   First, you teach something like, “Bigger bottom borrows” to help students decide when to borrow.  Then you prompt them to explain how they know whether or not to borrow.  All of that should be asked and answered in the right place and at the right time.  A script or a posted process chart will help you remember all the decisions that have to be made, and what to look at to make the right one.  Without a script it is very unlikely that you will remember the exact wording each time.  You need a script to be able to deliver consistent language of instruction.

Math teaching strategy: Plan ahead to carefully choose the right examples. 

With some math procedures it is quite hard to choose the right examples.  The fine points can be obscured when the examples the teacher happens to come up with, are not quite right.  The examples may be an exception or handled differently in a way the procedure has not taught.  So for example borrowing across a zero is different than across other numerals so the numbers in a minuend must be chosen carefully rather than off the cuff.

Also, when teaching a procedure it is essential to teach when to use the procedure and when not to use that procedure.  It is important that the teacher present “non-examples,” that is, problems in which you don’t follow that procedure.  I have seen students who are taught, for example, borrowing, using only examples that need borrowing.  Then they turn around and borrow in every problem–because that is what they were taught.  They should have been taught with a few non-examples mixed in, that is, problems where borrowing wasn’t necessary so they learned correctly when to borrow as well as how to borrow.   Choosing teaching examples on the fly will often end up with more confusion rather than less.

If it bothers you to see students as frustrated as the one above, then find* or write out a script for teaching computation so that you can be consistent and effective.  Trust me, your students will love you for it.

* You may want to look at the “Learning Computation” programs within the Rocket Math Universal subscription.  Here are links to blogs on them:  Addition, Subtraction, and Multiplication.  These are sensible, small steps, clearly and consistently scripted so each skill builds on the next.

Math Teaching strategy #4: Teach only one procedure at a time

Posted on by Dr. Don

It’s far better to know only one way to get there, than to get lost every time!

There are educational gurus out there promoting the idea that by giving students multiple solution paths it will give them a deeper understanding of math.  Generally these experts know this from teaching pre-service teachers in college, some of whom come to have some insights by learning multiple paths to the same goal.  Sorry folks.  What works for pre-service teachers in college, does not [and never will] apply to most children.

True, there are multiple ways to solve most arithmetic problems.  They have been discovered over centuries across multiple civilizations.  While one might dream of knowing all the ways to do long division, it’s far better to have one reliable method learned than to simply be confused and to get lost each time.  Just as in directions to go someplace, it is hard to remember all the steps in the directions.  When you’re new to a destination, the lefts and the rights are all arbitrary.  If you get two or more sets of directions, you are going to mix the steps from one way with the steps from the other method and you will not arrive at your destination.

Math teaching strategy: Teach one solution method and stick to that until everyone has it mastered.

In real life, as in math, once you learn one reliable method of getting to your destination, you are then free to learn additional ways, or to try short cuts.  But please don’t confuse a beginning learner with short cuts or alternative methods.  It adds to the memory load and there are additional things to think about when trying alternatives.  Before the learner is solid in one method, the new information is likely going to get mixed up with the not-yet-learned material, leading to missteps and getting lost. Teach the long way every time and leave them to finding the short cuts on their own time.

But teachers say, “I want them to have a holistic understanding of what they are doing!”  Which is laudable, but that understanding has to come AFTER a reliable set of procedures is mastered.  There’s no reason that additional learning can’t be added to the student’s knowledge base, but it can’t come before or in place of learning a simple, basic, reliable procedure.   These admirable goals of getting a deeper understanding of math are fine, but they require MORE teaching than what used to be done, back when we were only taught algorithms for arithmetic.  There is time to learn more than the algorithms, if we teach effectively and efficiently.  Unfortunately, the deeper and more profound understandings in math can’t precede or be substituted for teaching the algorithms.

If you don’t believe me, ask a typical middle school student to do some arithmetic for you these days.  Few of them have mastered any reliable procedures for doing long division or converting mixed numbers or adding unlike fractions, etc.  It’s time to accept that teaching one way of doing things is better than none.

Math Teaching strategies #5: Separate the introduction of similar concepts

Posted on by Dr. Don

Teaching two similar concepts and their vocabulary terms at the same time creates confusion.

The classic example is teaching parallel and perpendicular on the same day.  The two concepts have to do with orientation of lines and the new vocabulary terms for them are similar.  So teaching them at the same time means some or many students will have the two terms confused for a long time.  That is known as a chronic confusion–possibly permanent.  They will know that orientation of lines is one of those two terms, possibly, but will be confused about which is which.  The predictable conversation with the teacher goes thusly:

Teacher:  See these two lines lines.  Are they parallel or perpendicular?

Student: I think they’re perpendicular.

Teacher: Well…

Student: No, wait! I know.  They’re parallel.

Teacher: Yes, you’re right.  I’m glad you’ve learned that.

In case you missed it, the above student did NOT know the correct term.  The student just knew it was one of two terms, but unsure as to which one.  So the student picked one and as soon as there wasn’t confirmation by the teacher, switched to the other term.  When you’re busy teaching it is easy to get fooled by that kind of response into assuming the students really did learn it.

Other examples abound in math.  Teaching numerator and denominator in the same lesson is common.  Teaching the terms proper fractions and improper fractions on the same day is another example.  Acute and obtuse angles are yet another pair of chronically confused concepts that are introduced simultaneously.

Math teaching strategies: Separate the introduction of similar concepts in time.

If you teach one concept and only one of the pair, there’s no cause for confusion.  It will still take a lot of repetition and practice for it to be cemented into memory, but students will be clear.  If they use the term, for example parallel a lot of times in conjunction with examples they will soon (in a couple of weeks) be able to recall.  However, you should pair the concept with non-examples of the concept.  Not the opposite necessarily but just not an example of the concept.

For example: For the picture to the right you would ask the students.

A “Are the two lines in item A parallel or not parallel?”   Ans: Not parallel.

B “Are the two lines in item B parallel or not parallel?”   Ans: Parallel.

C “Are the two lines in item C parallel or not parallel?”   Ans: Not parallel.

Then after a couple of weeks you could introduce perpendicular.  Again teach it on its own and then contrasted with non-examples until the vocabulary was clear. Probably for a couple of weeks.   Only then can you combine both terms in the same lesson.

Another advantage of this approach is that you have avoided the other common mistake.  Students sometimes come to the conclusion that all pairs of lines are either parallel or perpendicular.  This method of introducing the concepts helps them realize that there are non-examples of each concept, parallel lines and ones that aren’t as well as perpendicular lines and ones that aren’t as well.

 

Math teaching strategies #6: Teaching a new concept using a common sense name

Posted on by Dr. Don

 

Here’s an example of the problem.   A brachistochrone (pictured above) is a curve between two points along which a body can move under gravity in a shorter time than for any other curve. It is the same curve as a cycloid, but just hanging downward.   A cycloid is the path traced by a point on a wheel as the wheel rolls, without slipping, along a flat surface. The standard parametrization is x = a(t – sin t),y = a(1 – cos t), where a is the radius of the wheel.

Introducing this concept and the term brachistochrone at the same time would be designed so the teacher can use brachistochrone and its concept in instruction.  However, because the term is new and the concept is also new, when the teacher uses the new term during later instruction, the students will have difficulty bringing the concept into their minds. “So a brachistochrone has some other cool properties. What’s the primary thing we know about the brachistochrone?”

Instruction not working.  If you watch instruction where the term and the concept have been taught simultaneously, confusion ensues when the teacher uses the term.  You’ll see students looking away as they try to bring up the explanation of that weird new term in their memory (see the pictured example?).  Sometimes the teacher will notice this and give a thumbnail definition or example of the term, and the students will then remember.  However, the teacher should then realize that the concept was not connected to the new vocabulary term.

Math teaching strategies: Teach new concepts using a common sense term at first.  

When a new vocabulary term is used to introduce a new concept, students will need a lot of practice with recalling the term and the definition before it can be used in instruction.  On the other hand, students can quickly understand and use new concepts and ideas in math if they don’t have to learn a new word for it.  Using a common sense term, the idea or concept can be worked with, the implications studied and it can be applied to real world problems almost immediately.  Students can later quite easily learn proper vocabulary terms for concepts they understand and recognize.  Here’s an example. 

The “shortest time curve.”  It is more efficient and effective to teach the concept first and use a common sense term for it.  I would call a brachistochrone the “shortest time curve.”**  Instruction would proceed with the, “Do you remember that ‘shortest time curve’ we talked about last week?”  Students would easily be able to remember it.  Instruction would go like this: “So ‘the shortest time curve’ has some other cool properties. What’s the primary thing we know about the ‘shortest time curve’?”  Students would easily be able to answer this question.

Then after students have worked with the concept of “the shortest time curve” for a couple of weeks, you can add the vocabulary term to it. “By the way, the proper mathematical name for “the shortest time curve” is called a brachistochrone. Isn’t that cool?”  Students will want to learn its proper name as a point of pride about knowing this fancy term for a concept they already “own,” rather than a point of confusion.

**Actually that’s what brachistochrone means in Greek: brakhistos, meaning shortest and khronos meaning time.